Difference between revisions of "Data Management"
Pthomadakis (talk | contribs) (→Particle Trajectory Tracking with ML) |
Pthomadakis (talk | contribs) (→Particle Trajectory Tracking with ML) |
||
| Line 42: | Line 42: | ||
== Particle Trajectory Tracking with ML == | == Particle Trajectory Tracking with ML == | ||
[[CNF:Machine_Learning | Machine Learning for Track Classification and Prediction]] | [[CNF:Machine_Learning | Machine Learning for Track Classification and Prediction]] | ||
| + | |||
[[CNF:ML_Data_Denoise | Machine Learning for Track Denoising]] | [[CNF:ML_Data_Denoise | Machine Learning for Track Denoising]] | ||
Latest revision as of 23:35, 3 November 2020
Contents
- 1 Next-generation imaging filters and mesh-based data representation for phase-space calculations in nuclear femtography
- 2 Telescopic Approach
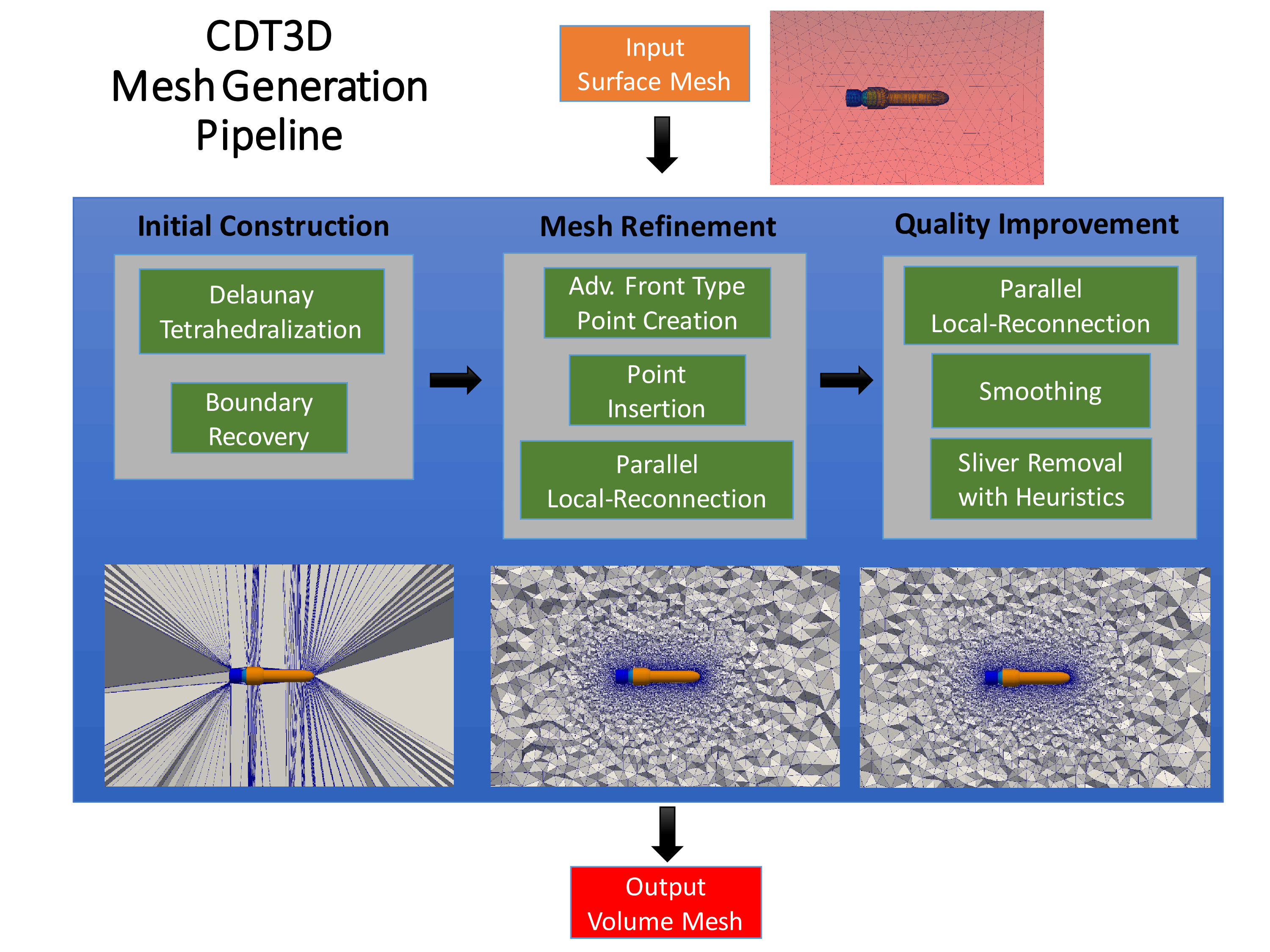
- 3 Exascale-Era Finite Element Mesh Generation
- 4 Boundary Recovery
- 5 Isotropic Mesh Generation
- 6 Anisotropic Mesh Generation
- 7 Runtime Systems
- 8 Particle Trajectory Tracking with ML
Next-generation imaging filters and mesh-based data representation for phase-space calculations in nuclear femtography
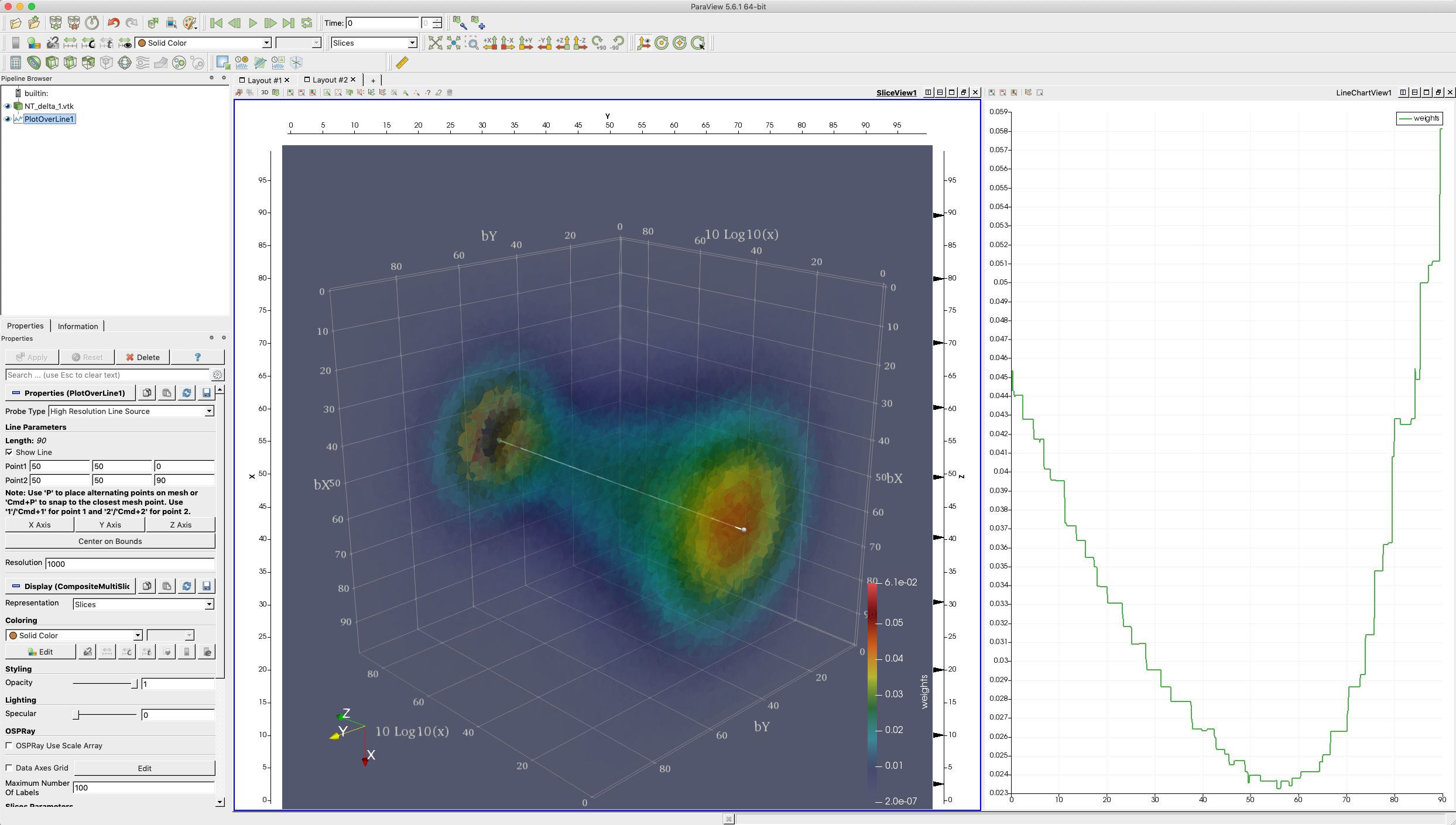
- Tomographic pictures of the nucleon
Tomographic and recently aquired and tessellated pictures of the nucleon as a result of this project. Namely, the plots show a spatial distribution of up quarks as a function of proton's momentum fraction carried by those quarks. Specifically, bX and bY are the spatial coordinates (in 1/GeV = 0.197 fm) defined in a plane perpendicular to the nucleon’s motion, x is the fraction of proton’s momentum and color denotes probability density for finding a quark at given (bX, bY, x).
Plots produced by Dr. Gagik Gavalian and Dr. Pawel Sznajder and tesselated by CRTC's Image-to-Mesh (I2M) conversion software deployed to Jefferson Lab last month.
For more data and information about this project follow this link: CNF
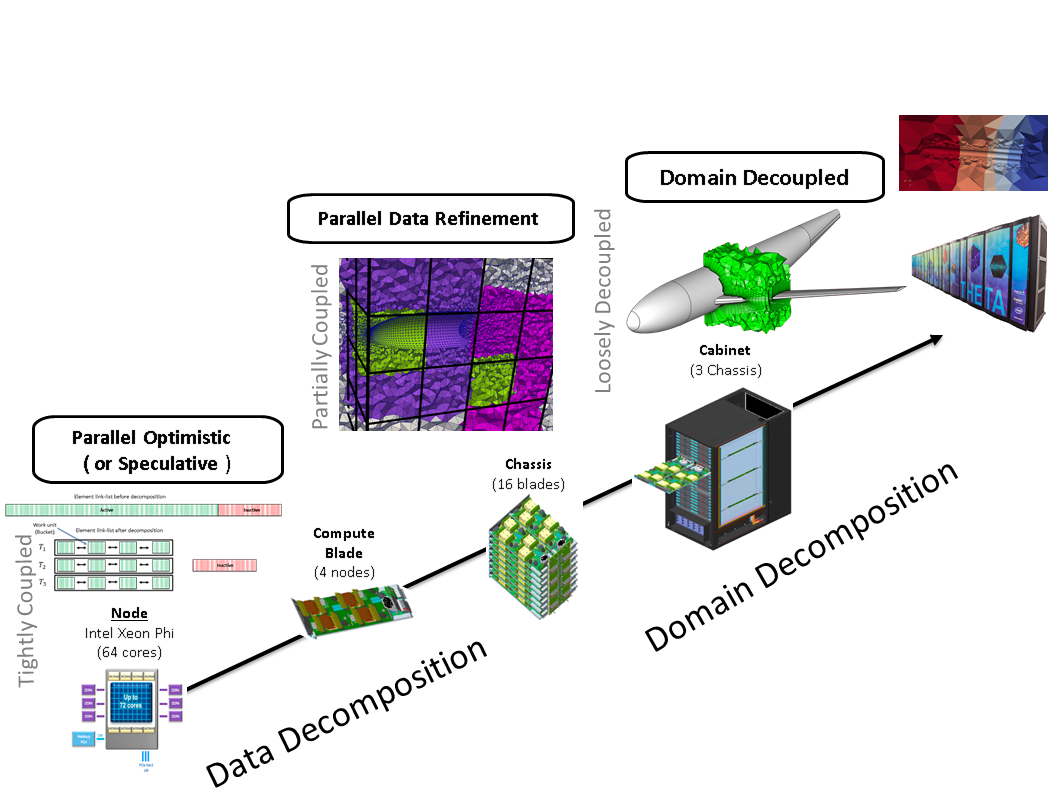
Telescopic Approach
- The telescopic approach of CRTC lab
The project aims at investigating the design and implementation of multi-layered algorithmic and software framework for 3D tetrahedral parallel mesh generation. The framework is referred as the Telescopic Approach
Exascale-Era Finite Element Mesh Generation
